Statistiche del giocatore

2 460 101 881

Ottieni crediti gratis!

Invita nuovi utenti ad iscriversi al gioco e otterrai 12 crediti per ciascun referral!

Prossima partita

Axoloth Griffins

Partita di lega
26.2.2025 18:00

HRS

MIN

SEC

Questa funzione fa parte del pacchetto PRO!

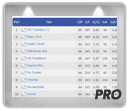
Versione PRO della pagina delle statistiche di Lega

Nella pagina delle statistiche di lega avete la possibilità di passare alle statistiche PRO per poter vedere maggiori dati come i goal in power play e in inferiorità numerica o il numero di shutout dei portieri.

Attiva il PRO pack

Acquista crediti

Vantaggi del pacchetto PRO

Pro

I giocatori migliori alla fine della regular season ricevereanno un bonus nella loro popolarità e i loro club riceveranno una ricompensa finanziaria dai commissari della lega. Le statistiche dei playoff includono i quarti di finale, le semifinali, le finali e la partite di piazzamento per il terzo posto.

Stagione: Nazione: Lega:

Giocatori

Pos	Nome	Squadra	GP	G	A	TP	+/-	PIM	S	S%	3SS	AS
1	Marc Tukker	Team noname 402	7	4	15	19	8	0	13	30.8	2	0
2	Koen Aarninkhoff	Team noname 205	9	7	11	18	2	0	23	30.4	3	0
3	Niek Beekman	Team noname 402	7	5	13	18	8	0	10	50.0	2	0
4	Wessel Mulders	Team noname 402	7	10	7	17	8	4	19	52.6	3	0
5	Jorian Dresen	Team noname 478	6	8	9	17	13	2	16	50.0	3	0
6	Bram Kaltenbach	Team noname 205	7	6	10	16	7	2	17	35.3	2	0
7	Pim Camphens	Team noname 205	7	5	11	16	9	2	7	71.4	2	0
8	Daniël Wierdsma	Team noname 478	6	4	12	16	14	2	15	26.7	4	0
9	Jaap Bijlaart	Team noname 402	8	2	14	16	1	2	26	7.7	2	0
10	Aron Eshuijs	Team noname 205	9	9	6	15	1	6	25	36.0	1	0
11	Stephan Hemelaar	Team noname 402	8	7	8	15	-1	2	17	41.2	2	0
12	Gerjan Vogt	Team noname 478	6	6	9	15	14	2	21	28.6	3	0
13	Wilfred Röhner	Team noname 205	5	5	10	15	-4	0	12	41.7	1	0
14	Tom Beekhuijs	Team noname 205	7	5	10	15	-5	2	16	31.3	3	0
15	Henk van Eijkel	Team noname 402	8	10	4	14	1	4	23	43.5	1	0
16	Thomas Battem	Team noname 205	5	5	9	14	3	0	10	50.0	1	0
17	Hermen van Niers	Team noname 205	9	5	9	14	2	8	26	19.2	1	0
18	Joris Betzel	Team noname 402	8	2	12	14	-1	0	16	12.5	0	0
19	Merein Knip	Team noname 205	6	7	6	13	6	0	16	43.8	2	0
20	Bas d' Arnaud	Team noname 402	8	6	7	13	-1	2	19	31.6	2	0
21	Gerard Barstra	Team noname 205	7	5	8	13	-5	2	19	26.3	0	0
22	Matthijs Snoeijing	Team noname 205	9	5	8	13	1	6	18	27.8	2	0
23	Lukas Stegehuis	Team noname 205	9	4	9	13	1	6	20	20.0	2	0
24	Bas van der Zalm	Team noname 204	4	3	10	13	6	2	9	33.3	2	0
25	Corné Vrielijnck	Team noname 205	7	3	10	13	9	2	16	18.8	2	0
26	Dirk Carpreliaux	Team noname 205	9	3	10	13	-2	8	15	20.0	2	0
27	Hielke Vallee	Team noname 205	5	8	4	12	5	2	20	40.0	3	0
28	Hilco Ramlal	Team noname 204	5	8	4	12	5	2	19	42.1	2	0
29	Floor Masselin	Team noname 205	7	8	4	12	9	4	13	61.5	2	0
30	Mark Wools	Team noname 205	5	6	6	12	11	2	13	46.2	1	0
31	Peter Grevenga	Team noname 205	7	6	6	12	9	4	20	30.0	1	0
32	Nathaniël Esman	Team noname 551	7	6	6	12	5	0	18	33.3	2	0
33	Thijs Weststrate	Team noname 551	7	5	7	12	0	0	17	29.4	2	0
34	Nelis Feiken	Team noname 551	7	5	7	12	5	0	9	55.6	1	0
35	Kars de Vooght	Team noname 205	5	4	8	12	-4	0	13	30.8	1	0
36	Rutger Mölder	Team noname 402	8	4	8	12	0	0	17	23.5	1	0
37	Twan Paping	Team noname 205	5	3	9	12	-2	6	16	18.8	1	0
38	Bram van Mastenbroek	Team noname 205	5	6	5	11	-2	4	20	30.0	0	0
39	Yorick Bussemakers	Team noname 205	6	6	5	11	-8	2	21	28.6	1	0
40	Joran van de Grampel	Team noname 478	6	6	5	11	0	0	16	37.5	0	0
41	Wiard Linschooten	Team noname 205	5	5	6	11	11	6	17	29.4	1	0
42	Ethan Beijers	Team noname 205	6	5	6	11	6	0	18	27.8	1	0
43	Emirhan van Oostveen	Team noname 551	7	5	6	11	5	2	13	38.5	1	0
44	Rick de Waaij	Team noname 205	7	5	6	11	-5	2	11	45.5	0	0
45	Henry Nellen	Team noname 205	9	5	6	11	1	2	18	27.8	1	0
46	Anne Bregten	Team noname 205	4	4	7	11	2	0	12	33.3	2	0
47	Gerrit de Jeger	Team noname 205	7	4	7	11	9	4	16	25.0	0	0
48	Wessel Hetterschijt	Team noname 205	7	4	7	11	-5	2	14	28.6	0	0
49	Anish van Hunnik	Team noname 551	7	4	7	11	0	0	12	33.3	1	0
50	Peter Feldmeijer	Team noname 205	5	3	8	11	11	4	13	23.1	1	0

Portieri

Pos	Nome	Squadra	GP	TOI	SA	GA	Sv%	3SS	AS
1	Jelmer Plats	Team noname 478	6	360	158	28	82.28	0	0
2	Maarten Mannheim	Team noname 205	5	300	149	29	80.54	0	0
3	Isa van Hoof	Team noname 204	4	150	64	13	79.69	0	0
4	Bernard Stockinger	Team noname 205	6	360	176	39	77.84	0	0
5	Jeffrey van Es	Team noname 551	7	425	183	41	77.60	0	0
6	Geert Sikkemeijer	Team noname 205	7	420	191	48	74.87	0	0
7	Corné Heineken	Team noname 402	8	480	238	61	74.37	0	0
8	Twan Maeghs	Team noname 205	9	540	231	61	73.59	0	0
9	Sander van der Veen	Team noname 733	4	240	116	31	73.28	0	0
10	Tim Kopjes Niemann	Team noname 205	5	305	148	40	72.97	0	0
11	Willem Zellner	Team noname 205	6	365	178	50	71.91	0	0
12	Dennie Verstappen	Team noname 402	7	420	179	51	71.51	0	0
13	Arjen van Ris	Team noname 205	4	247	94	29	69.15	0	0
14	Peter Goor	Team noname 204	5	300	132	43	67.42	0	0
15	Maurice Cordesius	Team noname 205	5	302	147	49	66.67	0	0
16	Ferry Schilperoort	Team noname 733	6	370	171	59	65.50	0	0
17	Vincent Beentjes	Team noname 204	4	100	46	17	63.04	0	0